Continuing with the applications of physics to the guitar:

Vibrato, like bending, is a staple in every lead guitarist's arsenal. It is very similar to bending, as a matter of fact, and is basically just bending up and down repeatedly to alter the frequency.

Last post, I ended with a monster equation used to determine the frequency of a bent string as it relates to a bend angle theta. This equation was derived by physicist David Robert Grimes who did an excellent job of condensing these techniques down into comprehensible equations. Here's the bend frequency equation again: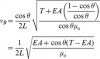

So, to find the rate of change of frequency caused by vibrato, we'll need an all-new equation. How do we deal with rates of change in equations? Well, we describe them using derivatives. Therefore, to find the rate of change of frequency brought on by constant vibrato, the derivative of both sides of the equation was taken: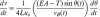

Keep in mind that the (t) following some of these terms just means that the terms are functions of time.

We can see at the very least the relationship between the derivative of the angle with respect to time...as dθ/dt increases, dv/dt increases. dθ/dt may look familiar, and that's because it's the angular velocity equation - so we can mathematically prove something that common sense would already have us believe. If one were to bend the string up and down faster and faster, the frequency would also change faster and faster.

But as every guitar player knows, it is way harder to bend an acoustic guitar's strings, or a classical guitar's, than it is to bend the string of an electric guitar. Therefore, since the bend angles on classical guitars are exceedingly small, Grimes developed a specialized equation specifically for them. This equation relates not the bend angle with frequency changes, but the tension in the string, as a classical guitar's strings are mostly bent using varying tensional forces.

Time for an example to break up the monotony:

When the guitar kicks in around 2:08 until the end of this intro part is full of vibrato. It's also awesome. One of the best guitar songs of all time.

Citation:

Grimes, David R. "String Theory - The Physics of String-Bending and Other Electric Guitar Techniques." PLOS ONE:. N.p., 23 July 2014. Web. 23 Nov. 2014.