Tag Archives: vectors
Victory over Vectors SimuLAB
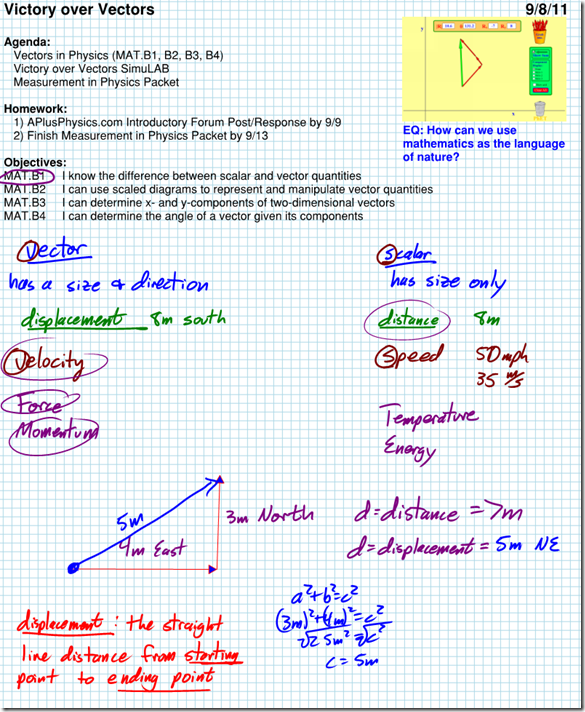
Vector Manipulation
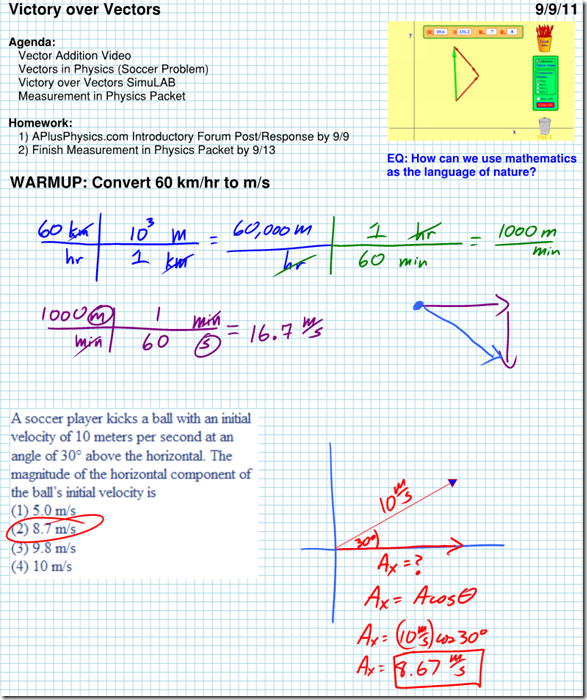
Quantities in physics are used to represent real-world measurements, and therefore physicists use these quantities as tools to better understand the world. In examining these quantities, there are times when just a number, with a unit, can completely describe a situation. These numbers, which have a magnitude, or size, only are known as scalars. Examples of scalars include quantities such as temperature, mass, and time. At other times, a quantity is more descriptive if it also includes a direction. These quantities which have both a magnitude and direction are known as vectors. Vector quantities you may be familiar with include force, velocity, and acceleration.
Most students will be familiar with scalars, but to many, vectors may be a new and confusing concept. By learning just a few rules for dealing with vectors, though, you’ll find that they are a powerful tool for problem solving.
Vectors are often represented as arrows, with the length of the arrow indicating the magnitude of the quantity, and the direction of the arrow indicating the direction of the vector. In the figure at right, vector B has a magnitude greater than that of vector A. Vectors A and B point in the same direction, however. It’s also important to note that vectors can be moved anywhere in space. The positions of A and B could be reversed, and the individual vectors would retain their values of magnitude and direction. This makes adding vectors very straightforward!
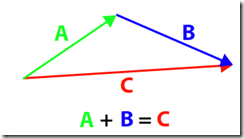To add vectors A and B, all we have to do is line them up so that the tip of the first vector touches the tail of the second vector. Then, to find the sum of the vectors, known as the resultant, all we have to do is draw a straight line from the start of the first vector to the end of the last vector. This method works with any number of vectors.
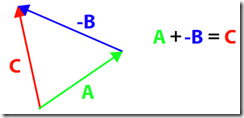
So then, how do we subtract two vectors? Let’s try it by subtracting B from A. We could rewrite the expression A – B as A + -B. Now it becomes an addition problem, we just have to figure out how to express –B. This is easier than it sounds – to find the opposite of a vector, we just point the vector in the opposite direction. Therefore, we can use what we already know about the addition of vectors to find the resultant of A–B.
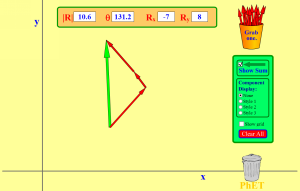
Components of Vectors
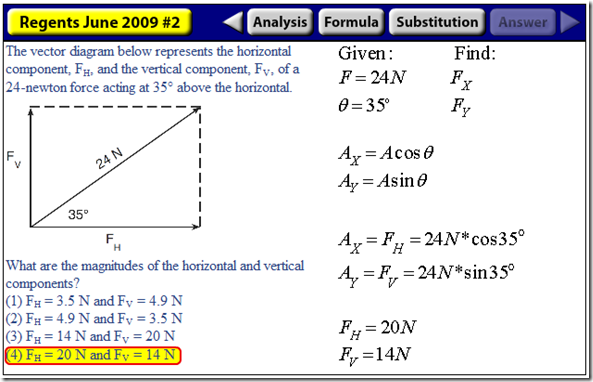
We’ll learn more about vectors as we go, but before we move on, there’s one more skill we need to learn. Vectors at angles can be challenging to deal with. By transforming a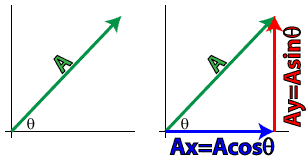
vector at an angle into two vectors, one parallel to the x-axis and one parallel to the y-axis, we can greatly simplify problem solving. To break a vector up into its components, we can use our basic trig functions. To help us out even further, the Regents Physics Reference Table includes the exact formulas we need to determine the x- and y-components of any vector if we know that vector’s magnitude and direction.
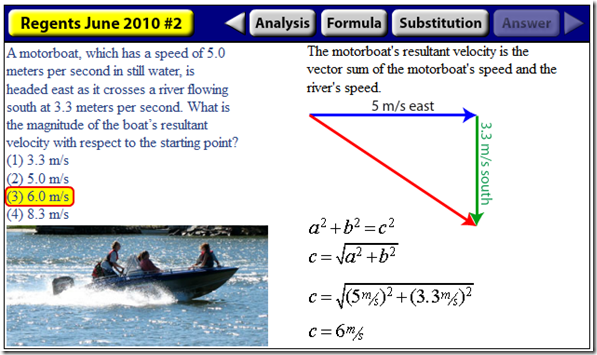
In similar fashion, we can use the components of a vector in order to build the original vector. Graphically, if we line up the component vectors tip-to-tail, the original vector runs from the starting point of the first vector to the ending point of the last vector. To determine the magnitude of the resulting vector algebraically, just apply the Pythagorean Theorem!
Intro to Vectors
Victory over Vectors PHET Simulation
| Click to Run |
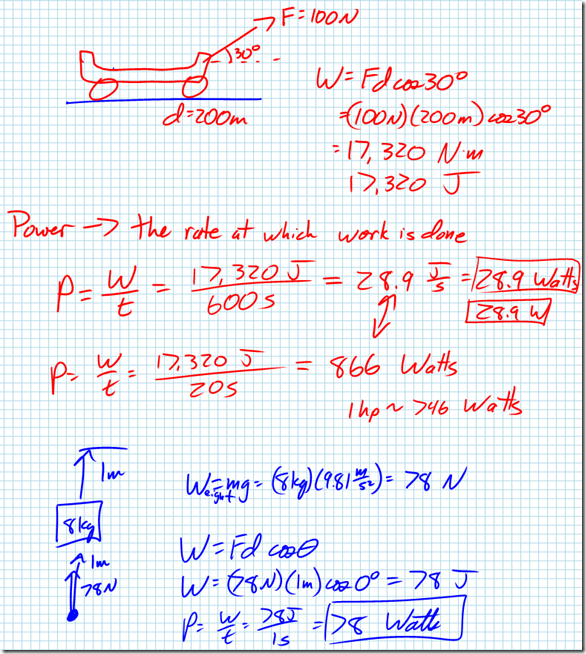
Intro to Work and Power
Defining Work
Sometimes we work hard. Sometimes we’re slackers. But, right now, are you doing work? And what do we mean by work?
Work — the process of moving an object by applying a force
In order for a force to qualify as having done work on an object, there must be a displacement and the force must cause the displacement.
I’m sure you can think up countless examples of work being done, but a few that spring to my mind include pushing a snowblower to clear the driveway, pulling a sled up a hill with a rope, stacking boxes of books from the floor onto a shelf, and throwing a baseball from the pitcher’s mound to home plate.
Let’s try an exercise: Which of the following are examples of work being done?
- Sandy struggles to push her stalled car, but can’t make it move.
- Jeeves the butler carries a tray above his head by one arm across the room at a constant velocity.
- A missile streaks through the upper atmosphere.
Each of these examples help us better understand the definition of work. In example 1, even though Sandy pushes her car, with all her might, the car doesn’t move, therefore no work is done.
Example 2 is a very tricky situation. In this scenario, Jeeves applies a force upward with his arm, but the tray moves horizontally. From this perspective, the force of the butler’s arm isn’t causing the displacement, therefore you could say no work is done by his arm. However, Jeeves’ legs are pushing him forward, and therefore the tray moves horizontally, so you could say from this perspective he is doing work on the tray. (In actuality, the situation is even more complex than this as we pull friction and normal forces into the equation, but for the sake of clarity, let’s move on…)
In example 3, the missile’s engines are applying a force causing it to move. But what is doing the work? The hot expanding gas is pushed backward out of the missile’s engine… so, using Newton’s 3rd Law, we observe the reactionary force of the gas pushing the missile forward, causing a displacement. Therefore, the expanding exhausted gas is doing work on the missile!
Calculating Work
Mathematically, work can be expressed by the following equation:
Where W is the work done, F is the force applied, in Newtons, and d is the object’s displacement, in meters.
The units of work can be found by a unit analysis of the work formula. If work is force multiplied by distance, the units must be the units of force multiplied by the units of distance, or newtons multiplied by meters. A newton-meter is also known as a Joule (J).
It’s important to note that when using this equation, only the force applied in the direction of the object’s displacement counts! This means that if the force and displacement vectors aren’t in exactly the same direction, you need to take the component of force in the direction of the object’s displacement. To do this, line up the force and displacement vectors tail-to-tail and measure the angle between them. Since this component of force can be calculated by multiplying the force times the cosine of the angle between the force and displacement vectors, we can re-write our work equation as:
Let’s examine a few more examples:
Question: An appliance salesman pushes a refrigerator 2 meters across the floor by applying a force of 200N. Find the work done.
Answer: Since the force and displacement are in the same direction, the angle between them is 0:
.
Question: A friend’s car is stuck on the ice. You push down on the car to provide more friction for the tires (by way of increasing the normal force), allowing the car’s tires to propel it forward 5m onto less slippery ground. How much work did you do?
Answer: You applied a downward force, yet the car’s displacement was sideways. Therefore, the angle between the force and displacement vectors is 90°, so:
.
Question: You push a crate up a ramp with a force of 10N. Despite your pushing, however, the crate slides down the ramp a distance of 4m. How much work did you do?
Answer: Since the direction of the force you applied is opposite the direction of the crate’s displacement, the angle between the two vectors is 180°.
Question: How much work is done in lifting an 8-kg box from the floor to a height of 2m above the floor?
Answer: It’s easy to see the displacement is 2m, and the force must be applied in the direction of the displacement, but what is the force? To lift the box we must match and overcome the force of gravity on the box. Therefore, the force we must apply is equal to the gravitational force, or weight, of the box:
Question:Barry, John, and Sidney pull a 30-kg wagon with a force of 500N a distance of 20m. The force acts at a 30° angle to the horizontal. Calculate the work done.
Answer:
Force vs. Displacement Graphs
The area under a force vs. displacement graph is the work done by the force. Consider the situation of a block being pulled across a table with a constant force of 5 Newtons over a displacement of 5 meters, then the force gradually tapers off over the next 5 meters.
The work done by the force moving the block can be calculated by taking the area under the force vs. displacement graph (a combination of a rectangle and triangle) as follows:
