Tag Archives: Ohm’s Law
Parallel Circuit Analysis
Another basic circuit type is the parallel circuit, in which there is more than one current path. To analyze resistors in a series circuit, we found an equivalent resistance. We’ll follow the same strategy in analyzing resistors in parallel.
Resistors in Parallel
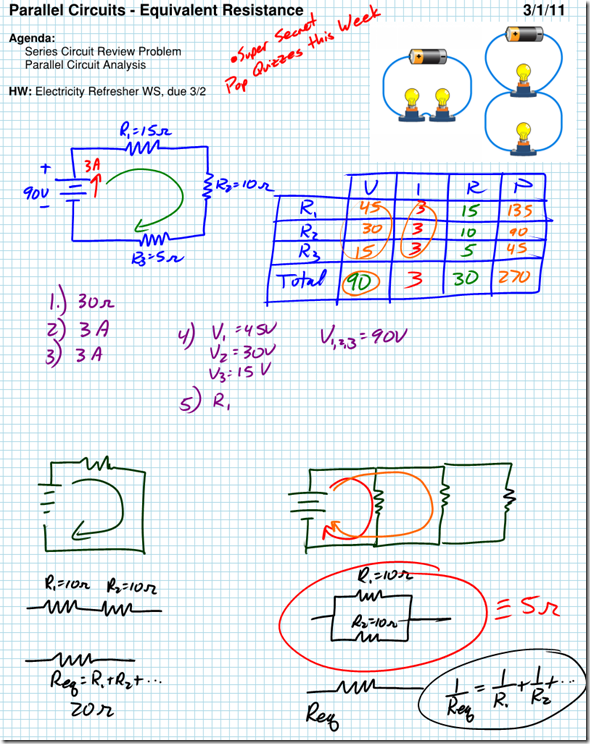
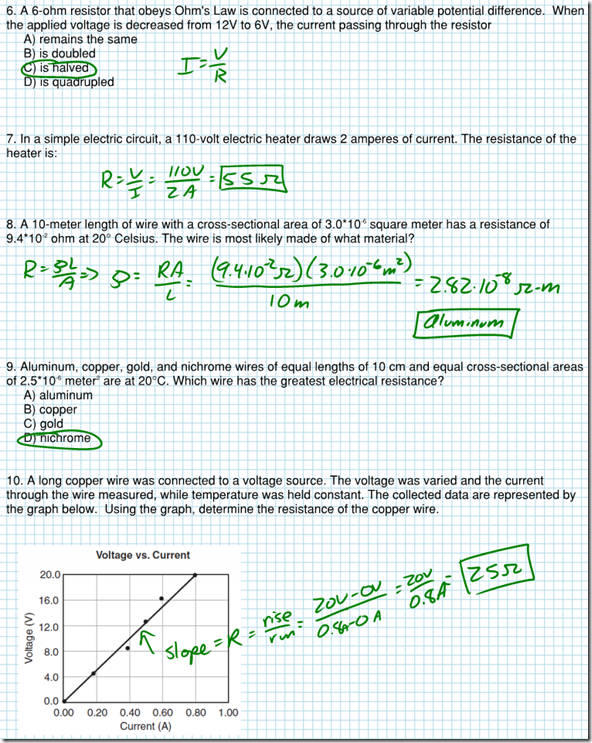
Let’s look at a circuit made of the same components we used in our exploration of series circuits, but now we’ll connect our components so as to provide multiple current paths, creating a parallel circuit.
Notice that in this circuit, electricity can follow one of three different paths through each of the resistors. In many ways, this is similar to a river branching into three different smaller rivers. Each resistor, then, causes a potential drop (analogous to a waterfall), then the three rivers recombine before heading back to the battery, which we can think of like a pump, raising the river to a higher potential before sending it back on its looping path.
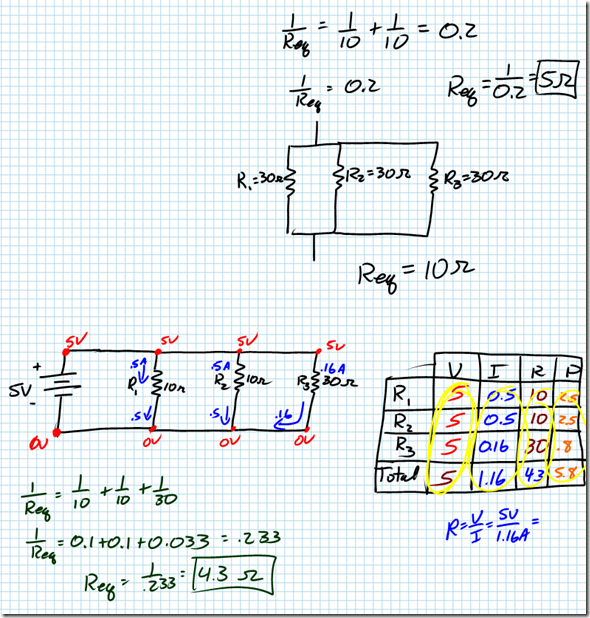
We can find the equivalent resistance of resistors in parallel using the formula:
![]()
Take care in using this equation, as it’s easy to make errors in performing your calculations. Let’s see if we can find the equivalent resistance for our sample circuit.

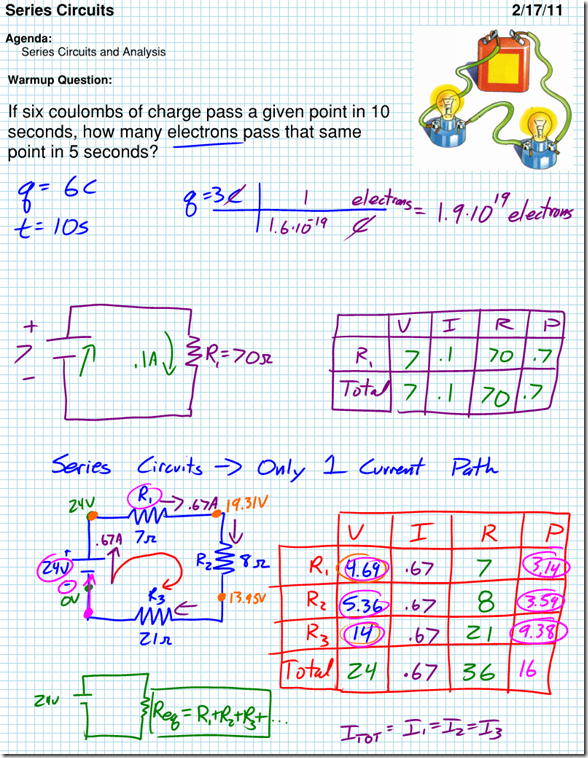
Series Circuits
Developing an understanding of circuits is the first step in learning about the modern-day electronic devices that dominate what is becoming known as the "Information Age." A basic circuit type, the series circuit, is a circuit in which there is only a single current path. Kirchhoff’s Laws provide us the tools in order to analyze any type of circuit.

Kirchhoff’s Laws
Kirchhoff’s Current Law (KCL), named after German physicist Gustav Kirchhoff, states that the sum of all current entering any point in a circuit has to equal the sum of all current leaving any point in a circuit. More simply, this is another way of looking at the law of conservation of charge.
Kirchhoff’s Voltage Law (KVL) states that the sum of all the potential drops in any closed loop of a circuit has to equal zero. More simply, KVL is a method of applying the law of conservation of energy to a circuit.
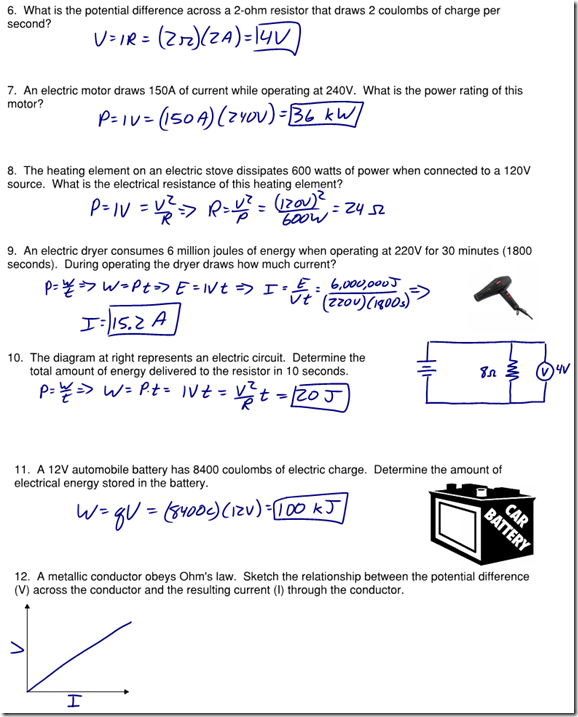
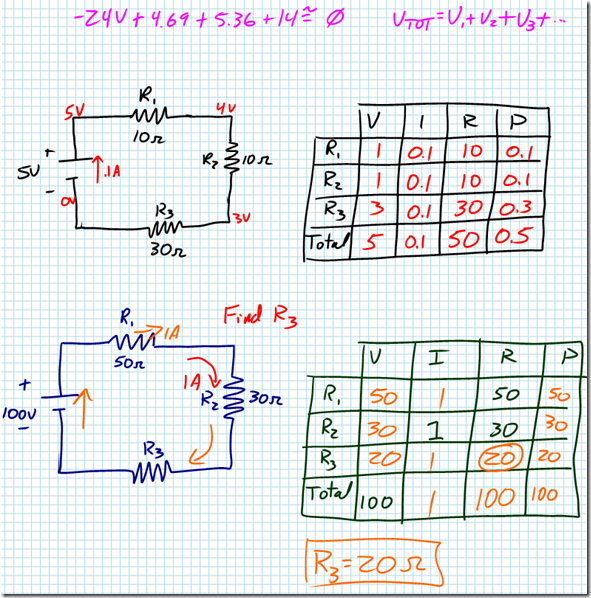
Question: A 3.0-ohm resistor and a 6.0-ohm resistor are connected in series in an operating electric circuit. If the current through the 3.0-ohm resistor is 4.0 amperes, what is the potential difference across the 6.0-ohm resistor?
Answer: First, let’s draw a picture of the situation. If 4 amps of current is flowing through the 3-ohm resistor, then 4 amps of current must be flowing through the 6-ohm resistor according to Kirchhoff’s Current Law. If we know the current and the resistance, we can calculate the voltage drop across the 6-ohm resistor using Ohm’s Law:
Resistors in Series
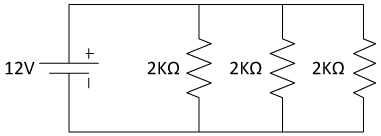
Let’s take a look at a sample circuit, consisting of three 2000-ohm (2K![]() ) resistors:
) resistors:
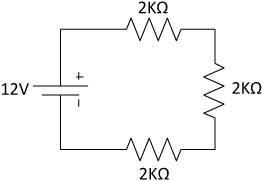
There is only a single current path in the circuit, which travels through all three resistors. Instead of using three separate 2K resistors, we could replace the three resistors with one single resistor having an equivalent resistance. To find the equivalent resistance of any number of series resistors, we just add up their individual resistances:
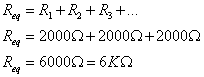
Note that because there is only a single current path, the same current must flow through each of the resistors.
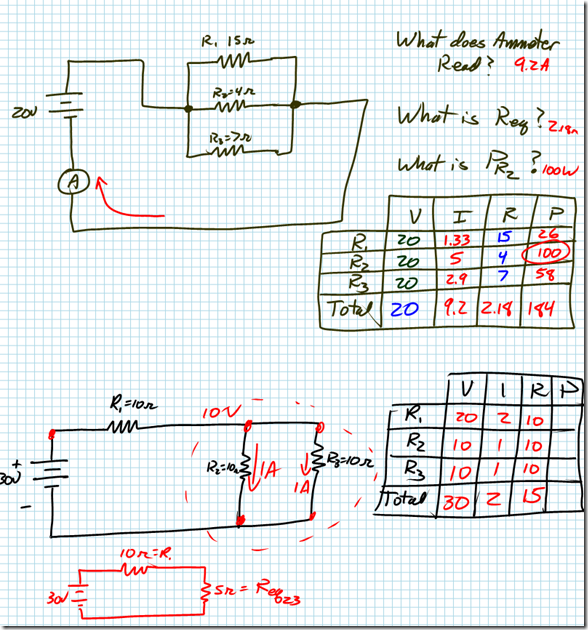
VIRP Tables
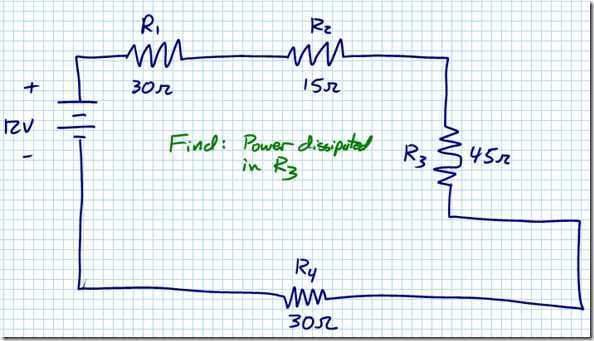
A simple and straightforward method for analyzing circuits involves creating a VIRP table for each circuit you encounter. Combining your knowledge of Ohm’s Law, Kirchoff’s Current Law, Kirchoff’s Voltage Law, and equivalent resistance, you can use this table to solve for the details of any circuit.
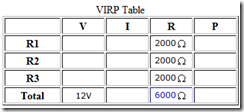
A VIRP table describes the potential drop (V-voltage), current flow (I-current), resistance (R) and power dissipated (P-power) for each element in your circuit, as well as for the circuit as a whole. Let’s use our circuit with the three 2000-ohm resistors as an example to demonstrate how a VIRP table is used. To create the VIRP table, we first list our circuit elements, and total, in the rows of the table, then make columns for V, I, R, and P:
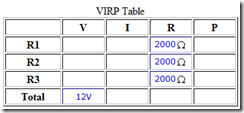
Next, we fill in the information in the table that we know. For example, we know the total voltage in the circuit (12V) provided by the battery, and we know the values for resistance for each of the individual resistors:
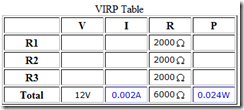
Once we have our initial information filled in, we can also calculate the total resistance, or equivalent resistance, of the entire circuit. In our case, this is 6000 ohms:
If I look at the bottom (total) row of my table, I know both the voltage drop (V) and the resistance (R). Knowing these two items, I can calculate the total current flow in the circuit using Ohm’s Law, and I can also calculate the total power dissipated in the circuit using my formulas for electrical power:

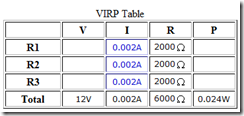
I can now fill in more information in the VIRP table:
Because this is a series circuit, the total current has to be the same as the current through each individual element, so I can fill in the current through each of the individual resistors:
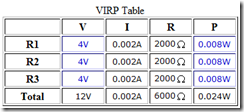
Finally, for each element in the circuit I now know the current flow and the resistance. Using this knowledge, I can apply Ohm’s Law to obtain the voltage drop (V=IR), and a formula for power (P=I2R) to complete the table.
So what does this table really tell us now that it’s completely filled out? We know the potential drop across each resistor (4V), the current through each resistor (2 mA), and the power dissipated by each resistor (8 mW). In addition, we know the total potential drop for the entire circuit is 12V, and the entire circuit dissipated 24 mW of power. Note that for a series circuit, the sum of the individual voltage drops across each element equal the total potential difference in the circuit, the current is the same throughout the circuit, and the resistances and power dissipated values also add up to the total resistance and total power dissipated. These are summarized for you on your reference table as follows: 
Disney Current and Resistance WS
Solutions: Resistance of a Wire WS
Ohm’s Law
Agenda:
- Ohm’s Law
- Ohm’s Law Lab
If resistance opposes current flow, and potential difference promotes current flow, it only makes sense that these quantities must somehow be related. George Ohm studied and quantified these relationships for conductors and resistors in a famous formula now known as Ohm’s Law:
![]()
Ohm’s Law may make more qualitative sense if we re-arrange it slightly:
![]()
Now it’s easy to see that the current flowing through a conductor or resistor (in amps) is equal to the potential difference across the object (in volts) divided by the resistance of the object (in ohms). If you want a large current to flow, you would require a large potential difference (such as a large battery), and/or a very small resistance.
Question: The current in a wire is 24 amperes when connected to a 1.5 volt battery. Find the resistance of the wire.
Answer:
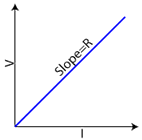
Note: Ohm’s Law isn’t truly a law of physics — not all materials obey this relationship. It is, however, a very useful empirical relationship that accurately describes key electrical characteristics of conductors and resistors. One way to test if a material is ohmic (if it follows Ohm’s Law) is to graph the voltage vs. current flow through the material. If the material obeys Ohm’s Law, you’ll get a linear relationship, and the slope of the line is equal to the material’s resistance.