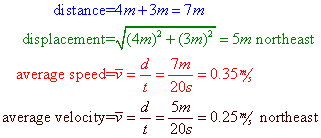Regents Physics - Defining Motion

Distance and Displacement

An object's position refers to its location at any given point in time. If we confine our study to motion in one dimension, we can define how far an object travels from its initial position as its distance, "d." Distance, as defined by physics, is a scalar. It has a magnitude, or size, only. The basic unit of distance is the meter (m).

Sample Problem:  On a sunny afternoon, a deer walks 1300 meters east to a creek for a drink.  The deer then walks 500 meters west to the berry patch for dinner, before running 300 meters west when startled by a loud raccoon.  What distance did the deer travel?

Answer: The deer traveled 1300m + 500m + 300m, for a total distance traveled of 2100m.

Besides distance, in physics it’s oftentimes helpful to know how far an object is from its starting point.  The vector quantity displacement describes how far an object is from its starting point, and the direction of the displacement vector points from the starting point to the finishing point.  Like distance, the units of displacement are meters.  Complicating matters, though, displacement also uses the same symbol as distance, a “d.”

Sample Problem: A deer walks 1300 m east to a creek for a drink.  The deer then walked 500 m west to the berry patch for dinner, before running 300 m west when startled by a loud raccoon.  What is the deer’s displacement?

Answer: The deer’s displacement was 500m east.

Notice how for the exact same motion, distance and displacement have significantly different values. Understanding the similarities (and differences) between these concepts is an important step toward understanding kinematics.

Speed and Velocity

Knowing only an object's distance and displacement doesn't tell us the whole story. Going back to our deer example, there's a significant difference in our picture of the deer's afternoon if the deer's travels occurred over 5 minutes (300 seconds) as opposed to over 50 minutes (3000 seconds).

How exactly does our picture change? In order to answer that question, we'll need to introduce some new concepts – average speed and average velocity. Both physics quantities use the same formula and the same units (m/s), but the symbols stand for different things in each formula. In this way, you can re-use the same formula to give you two different physics quantities. The formula, available on the back page of your reference table, is:

 

Again, notice how we get very different answers for average speed compared to average velocity… the difference is realizing that distance and speed are scalars, and displacement and velocity are vectors. One way to help you remember these: speed is a scalar, and velocity is a vector.

Sample Problem: Chuck the hungry squirrel travels 4m east and 3m north in search of an acorn.  The entire trip takes him 20 seconds.  Find: Chuck’s distance traveled, Chuck’s displacement, Chuck’s average speed, and Chuck’s average velocity.

Answer:

Let's try another sample problem to demonstrate the potential applications of these definitions.

Acceleration

So we’re starting to get a pretty good understanding of motion.  But what would our world be like if velocity never changed?  Objects at rest would remain at rest.  Objects in motion would remain in motion at a constant speed and direction.  And kinetic energy would never change (remember ?)  It’d make for a pretty boring world.  Thankfully, velocity can change in our world, and we call a change in velocity an acceleration.

More accurately, acceleration is the rate at which velocity changes.  We can write this as:

This indicates that the change in velocity divided by the time interval gives you the acceleration (another of our kinematic equations on the reference table). Much like displacement and velocity, acceleration is a vector – it has a direction. Further, the units of acceleration are meters per second per second, or [m/s²]. Although it sounds complicated, all the units mean is that velocity changes at the rate of one meter per second, every second. So an object starting at rest and accelerating at 2 m/s2 would be moving at 2 m/s after one second, 4 m/s after two seconds, 6 m/s after 3 seconds, and so on.

Of special note is the symbolism for ∆v.  The delta symbol (∆) indicates a change in a quantity, which is always the initial quantity subtracted from the final quantity.  For example:

Sample Problem: Monty the Monkey accelerates uniformly from rest to a velocity of 9 m/s in a time span of 3 seconds. Calculate Monty's acceleration.

Answer:

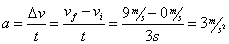
To develop another of our kinematic equations, we can combine the definition of acceleration () and the change in velocity equation () as follows:

 

Because acceleration is a vector and has direction, it's important to realize that positive and negative values for acceleration indicate direction only. Let's take a look at some examples. First, an acceleration of zero implies an object moves at a constant velocity. In the animation below, the car moves to the right with a constant velocity (zero acceleration).

Now, if we start the car at rest and give the car an acceleration to the right, the car speeds up as it moves to the right.

But what happens if we start the car with a velocity to the right, and give it an acceleration pointing to the left? The car will slow down as it moves to the right until its velocity becomes zero, then speed up as it continues accelerating to the left.

Positive accelerations don't necessarily indicate an object speeding up, and negative accelerations don't necessarily indicate an object slowing down. In one dimension, for example, if we call east the positive direction, a negative acceleration would indicate an acceleration vector pointing to the west. If the object is moving to the east (has a positive velocity), the negative acceleration would indicate the object is slowing down. If, however, the object is moving to the west (has a negative velocity), the negative acceleration would indicate the object is speeding up as it moves west.

Exasperating, isn't it? Putting it much more simply, if acceleration and velocity have the same sign (vectors in the same direction), the object is speeding up. If acceleration and velocity have opposite signs (vectors in opposite directions), the object is slowing down.